Hello! I’m Ste, and I’m researching voice AI at Gaudio Lab.

 

In the previous post, I talked about how Mahler’s dissonance was the language he used to express his emotions.
This time, I’d like to explore the traces and significance that this language left in the history of music.
Shall we dive deeper into Mahler’s music now? 🎶

 

 

2 Mahler

 

One of the composers who tackled this challenge was Mahler. In his symphonies, Mahler elevated dissonance to an artistic pinnacle. He once said, “A symphony must be like the world. It must embrace everything.” His music encapsulates the complexity of human emotions, the order and chaos of the universe, and the entirety of life and death. To Mahler, dissonance wasn’t merely a clash of notes; it was an essential tool that freely crossed the boundaries between harmony and tension, simultaneously expressing contradictory emotions.

 

In his symphonies, Mahler fused the diversity of the world into a cohesive whole, leading audiences toward new philosophical reflections. For instance, in Symphony No. 1, he sings of the vitality of spring while reflecting on his painful childhood, juxtaposing consonance and dissonance, harmony and chaos. In Symphony No. 2, he explores the journey from death to resurrection, expressing the weight of life and the possibilities beyond it.

 

Symphony No. 3 delves into the layers of existence, showing the harmony of nature, humanity, and love. The Adagietto of Symphony No. 5 is outwardly beautiful but imbued with the sorrow and anxiety of love, demonstrating how music can embody love, loss, pain, and joy simultaneously.

 

Mahler’s final symphony, Symphony No. 10, represents the zenith of his artistic exploration and showcases the climax of dissonance. Although unfinished, Mahler used this work to embrace pain and despair, transforming human wounds and suffering into an artistic universe through dissonance.

 

 

2.1 A Love Letter in the Key of A: The Fourth Movement of Symphony No. 5

 

The fourth movement of Mahler’s Symphony No. 5 is famously dedicated to his wife, Alma Mahler. Its distinctively beautiful melody is slow yet far from monotonous, moving listeners with its profound emotions. It has gained renewed attention recently, being featured in director Park Chan-wook’s film Decision to Leave, dramatically portraying the protagonist’s existential struggles between life and death.

 

Set in F Major, the movement is slow and tranquil, and the persistent use of non-chord tones evokes a reminiscent of Schumann’s piano piece Träumerei. Both share the same key, similar opening note structures, and the prominent use of a high A in their climactic sections. In Träumerei, this high A is sustained in the climax, initially harmonized with an A Major chord and later with a G Major 9th chord. The latter intensifies emotional tension as the A becomes the ninth, evoking poignant sentiments.

 

 

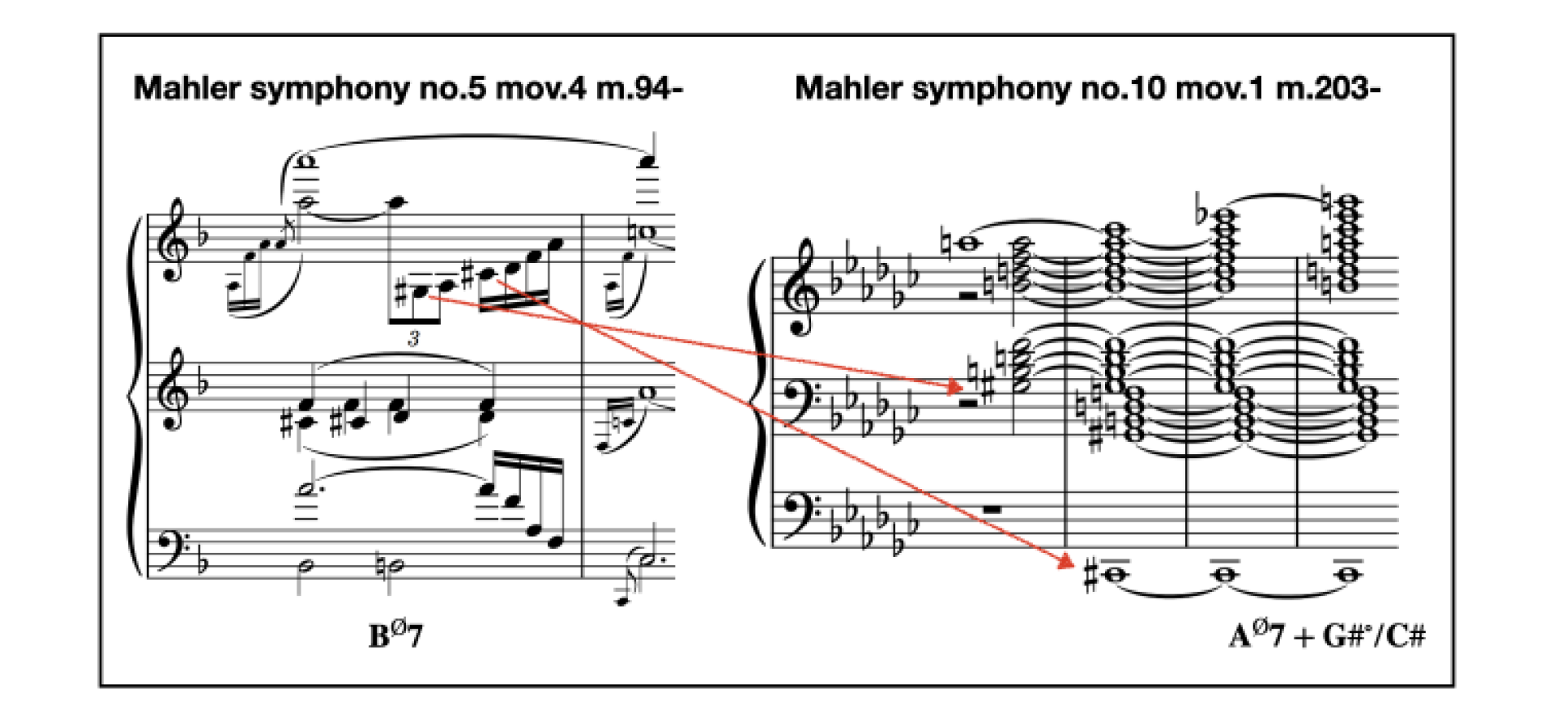
Figure 4: Excerpt from Mahler’s Symphony No. 5, 4th Movement (left) and Symphony No. 10, 1st Movement (right)

 

 

Mahler adopts a similar structure. The same high A note is harmonized with an F Major chord in the first section, while in the second section, as shown in Figure 4, it is embellished with a B♭mM7 chord, a Bø7 chord, and then resolves to an F Major chord. While transitioning through two chords to reach F Major, two non-chord tones are employed: G♯ moving toward A and C♯ resolving to D, played by the second violin.

 

 

2.2 “My Wife Has a Boyfriend”

 

Though Mahler and Alma were married, their love did not last. The reasons for their estrangement, as is often the case, remain known only to them—and sometimes not even to themselves. Alma, who had aspired to be a composer in her youth, abandoned her ambitions after marrying Mahler. Mahler once criticized her compositions, saying, “Her music is steeped in nauseating dilettantism, and her mind wanders lazily between fantasies of submission and domination.” [1]

 

However, Alma’s compositions, such as the first song in her 5 Lieder collection, Die Stille Stadt, reveal significant talent. The opening melody descends D-C-B♭-A-G, echoed in the piano accompaniment, which modulates the B♭. Her harmonic abilities—such as seamlessly transitioning from diminished seventh chords to different tonalities—demonstrate a solid musical education and an exceptional grasp of the floating harmony system prevalent in the late Romantic era.

Despite her evident talent, Mahler discouraged her from composing and harshly criticized her work. Why he did so remains unclear. During a period of separation, Alma had an affair with architect Walter Gropius. Gropius deliberately sent a letter intended for Alma to Mahler, revealing their relationship. Distraught, Mahler sought therapy from none other than Sigmund Freud. Though the details of their discussions remain confidential, it appears Freud’s counsel was effective to some extent. On his way home, Mahler wrote the following poem: [1]

 

 

 

 

Though Mahler often spoke harshly of his wife, his deep affection for her was undeniable. Despite receiving Alma’s promise to end her relationship with Gropius, their marriage never truly recovered. It was during this time that Mahler composed his final symphony, Symphony No. 10, pouring his inner torment into its notes.

The right-hand side of Figure 4 illustrates the intense dissonance in the symphony’s first movement. Mahler constructs an A∅7 chord anchored on the high A used in the climax of Symphony No. 5, juxtaposed against a G♯˚7 chord and further destabilized by a C♯ in the bass. These dissonant non-chord tones—G♯ and C♯—once enriched the expression of love in his earlier symphony but now create a wailing dissonance, mirroring the irreparable rift between Mahler and Alma. Unlike Mozart, who resolved dissonance consonance, Mahler leaves it unresolved, generating overwhelming beats akin to the tension in their estranged relationship.

 

 

3 And Beyond

 

At the turn of the 20th century, composers like Mahler began to incorporate dissonance and new harmonies more broadly into their works. Russian composer Stravinsky experimented with percussive dissonance in The Rite of Spring, while Hungarian composer Bartók fused folk melodies with unconventional harmonic elements in works like Music for Strings, Percussion, and Celesta. These approaches contrasted sharply with the lush tonal landscapes of Russian composer Rachmaninoff and the modal harmonies of French composers Debussy and Ravel.

 

Mahler’s exploration of dissonance influenced the next generation of Austrian and German composers. Arnold Schoenberg, for instance, developed the twelve-tone technique, which treated all twelve notes equally, laying the groundwork for serialism and influencing composers like Boulez and Stockhausen.

 

 

3.1 Penderecki’s Threnody to the Victims of Hiroshima

 

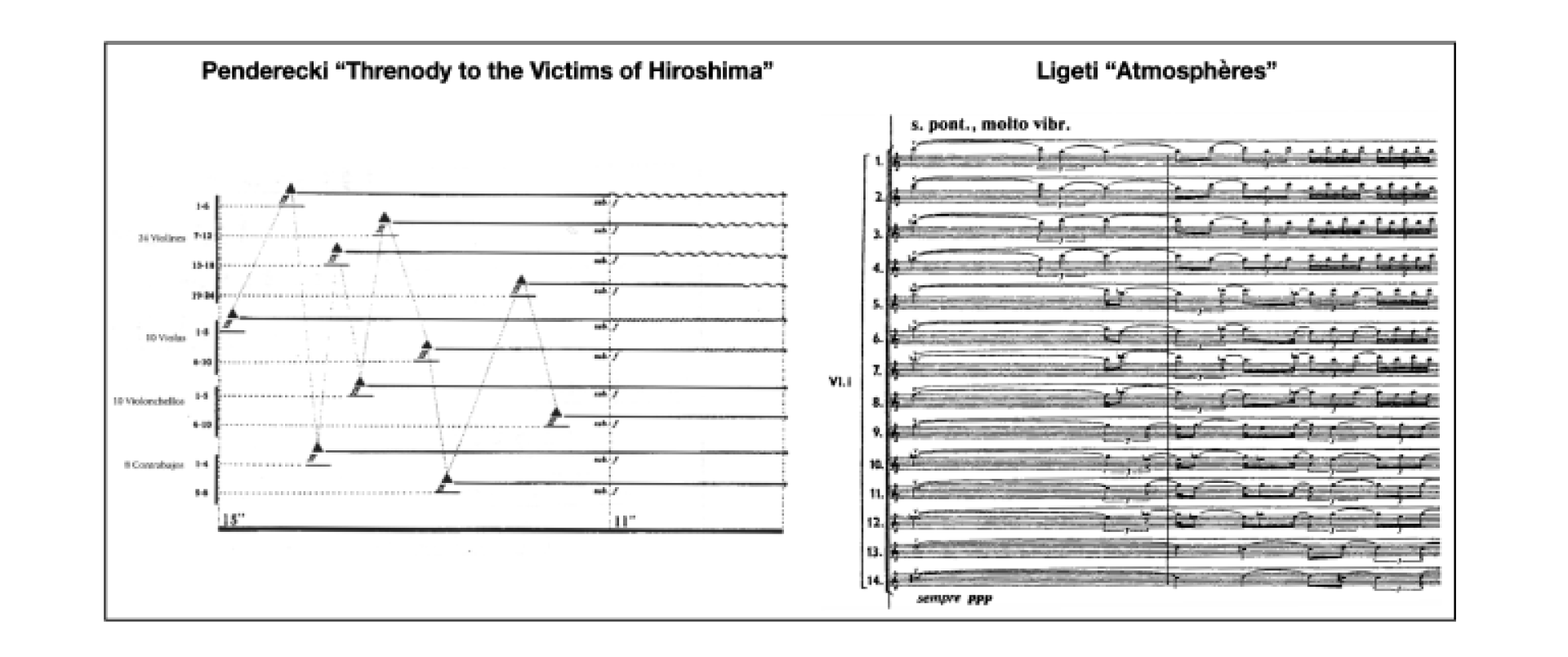
By the mid-20th century, clusters of dissonance had become a musical material in their own right, as seen in Penderecki’s Threnody to the Victims of Hiroshima. This piece, performed by a large string ensemble consisting of 24 violins, 10 violas, 10 cellos, and 8 double basses, opens with each instrument producing its highest possible note, played fortissimo. This piercing soundscape immediately immerses the listener in the raw, visceral emotions evoked by the aftermath of an atomic explosion. Penderecki employed not only traditional techniques like arco and pizzicato but also a wide range of modern techniques, including harmonics, col legno, and percussive effects such as tapping the instrument. These pushed traditional boundaries to deliver an unprecedented auditory experience. The result is an overwhelming cacophony that elicits a mix of terror and sorrow, perfectly encapsulating the devastating effects of the Hiroshima bombing.

 

 

3.2. Ligeti and Atmospheres

 

Hungarian composer Ligeti took a different approach, using dissonant tone clusters to create atmospheric effects in works like Atmospheres and Lux Aeterna. These pieces evoke the microscopic movements of molecules or photons, suggesting a detached beauty akin to background noise. Director Stanley Kubrick famously used these works in 2001: A Space Odyssey, pairing them with scenes of space’s stark, indifferent beauty.

 

Ligeti employed micropolyphony to achieve these effects, layering clusters of notes to create evolving textures. In Atmospheres, for example, a single sound unfolds over several minutes, shifting imperceptibly as it builds tension. Unlike Penderecki’s violent dissonance, Ligeti’s approach emphasizes subtlety, drawing the listener into a meditative and almost otherworldly state.

 

 

Figure 6: From Stanley Kubrick’s film 2001: A Space Odyssey

 

 

As I write this, a couple is arguing in the café behind me. The woman, having discovered the man’s infidelity, caused a commotion at his workplace. I don’t know who’s more at fault, but their quarrel feels like the tone clusters—simultaneously tragic and comedic. As the Korean pansori verse says:

 

 

“Oh, such is life—futile indeed.
Do you not see the peach blossoms in the Eastern Garden?
They bloom for but a moment.
Mistress of the brothel, why do you laugh?”

 

 

References

[1] Jens Malte Fischer. Gustav Mahler. Yale University Press, 2011.

[2] Hermann LF Helmholtz. On the Sensations of Tone as a Physiological Basis for the Theory of Music. Cambridge University Press, 2009.
[3] Reinier Plompand Willem JM Levelt. “Tonal consonance and critical bandwidth”. In: Journal of the Acoustical Society of America38(1965), pp. 548–560.